Energy Returned over Energy Invested (EROI) and Emergy

Table of Contents

 

Introduction

Balance Equations

Emergy in a Mark II Economy

The EROI Tables

The Effect of Lowering EROI toward 1.0.

Introduction

In http://dematerialism.net/Mark-II-Intro.html, of the four tasks achieved in this paper, the second and third were as follows:

2.   To eliminate the confusion regarding the ratio (EROI) of energy returned (ER) to energy invested (EI), five separate EROIs were defined depending upon what was included in the EI term.  The quantity EI^o includes only the direct energy costs of providing the net energy to the economy; EI¹ includes the indirect expenses but not those incurred by commerce; EI² includes, in addition, the  energy costs of commerce; EI³ includes, in addition, the energy costs of paying the managers in the energy sector more than the workers are paid; EI⁴ includes, in addition, the rest of the energy expenses of workers and managers in the energy sector and the pro-rata shares of the energy expenses of the workers and managers in other sectors insofar as they support the energy sector.  EROI^o is independent of political economy as the standard of living of the workers remains constant when the political economy changes.  Note: The interested reader should see http://dematerialism.net/eroeistar.htm for a better description of an all-inclusive energy-invested term.

3.  To illustrate graphically the effect of lowering the EROI due to substituting a less efficient technology for the technology that preceded it chronologically.  One sees the total energy budget, E, approaching infinity as EROI diminishes toward 1.0.  In this paper, I have defined Energy Returned to be the total energy extracted or produced.  This is the sum of the energy delivered and the energy invested.  An alternative definition counts only the energy delivered.  This has the advantage that it is easily computed in practice.  If the alternative definition is used, each of EROIs is reduced by exactly 1.0 and the total energy budget approaches infinity as EROI-1 approaches zero.  This result is illustrated graphically in Chart 1 on each of the two spreadsheets http://dematerialism.net/Mark-II-Economy.xls and http://dematerialism.net/Mark-II-Economy-CSP.xls.

Also, on Sheet 4 of both spreadsheets below and to the right of DN128, labeled Experiment 6, is a brief computation of emergy, which, in a Mark II Economy, is particularly simple as all energy is of the same type and may be assumed to be available energy with a transformity of 1.0.  The emergy of any energy product is the availability times the transformity as defined in http://dematerialism.net/Mark-II-Balance.html, in which energy, entropy, availability, and emergy balances for simple extraction and conversion processes for primary energy are derived.  The emergy of everything else if the sum of the emergy that went into producing it by an efficient process minus the emergies of any by-products that are produced.  If the emergies of the by-products are unknown, a canonical procedure for assigning them must be established possibly based upon market value, which is permissible because of the relation between emergy and money at any given time and place established in http://dematerialism.net/Mark-II-Money.html.

Balance Equations

Energy, entropy, availability, and emergy balances for simple extraction and conversion processes for primary energy are derived in http://dematerialism.net/Mark-II-Balance.html.  Some people may find these equations reassuring as far as the definitions of emergy and transformity are concerned.  Also, they illustrate exactly what is meant by energy returned over energy invested (EROI).  If enthalpy returned energy by HR and enthalpy invested by HI, entropy returned and invested by SR and SI, availability returned and invested by ER and EI, and emergy returned and invested by MR and MI, then

Clearly, by energy we refer, as usual, to availability.

Emergy in a Mark II Economy

Emergy is especially simple in a Mark II Economy because there is but one type of energy, and the transformity for that type of energy might as well be 1.0.  Thus, energy units can be taken to be emergy units of the primary energy.  Then, we can compute the emergy of each of the four commodities by “investing” one unit of each in the appropriate cell of DO31:DO34.  The change in the energy budget due to the consumption of one unit of each commodity that appears in DO27 is the emergy.  In Exercise 6 this was done and the results tabulated in DO130:DO133.  If each of these emergies is multiplied by the total consumption of the corresponding commodity, the sum of the products equals the total energy budget, which is not surprising.

The EROI Tables

On Sheet 4 of both spreadsheets five different EROIs for each political economy and for two levels of conservation are tabulated below and to the right of DN68:

The five types of EROI are as follows:

1.      EROI^o.  The energy invested (EI) is the direct energy overhead of the energy sector.

2.      EROI¹.  EI¹ includes, in addition to the direct energy overhead, the indirect energy costs associated with the energy overhead of the manufacturing and transportation portions of the overhead of the energy sector but not the overhead due to commerce.

3.      EROI².  EI² includes, in addition, the overhead due to the activities of commerce in connection with the sale of energy.

4.      EROI³.  EI³ includes, in addition, the consumption of energy associated with that portion of the salaries paid to the energy sector in excess of what they would have been if no one earned more than the workers do.  This is thought to account for over-consumption associated with profit taking in connection with the sale of energy.

5.      EROI⁴.  EI⁴ includes, in addition, the consumption of energy by the workers in the energy sector and the pro-rata shares of the energy expenses of the workers and managers in other sectors insofar as they support the energy sector.

The four political economies are as follows:

1.         The Base Case (BC) is a steady-state idealization of an American-style market economy.

2.         The No-Managers Case (NM) is a steady-state idealization of an American-style market economy with managers, presumably chosen by the workers from among themselves, that get paid the same as workers, which reduces energy consumption.  The expression No-Managers, then, is not particularly well-chosen.  I suppose this is an idealization of market communism.

3.         The No-Commerce Case (NC) is a planned economy that has a negligible energy  overhead but that has a commissar class which enjoys the same privileges as managers do in a market economy.  Perhaps it is an idealized Soviet economy.

4.         The No-Commerce-No-Managers Case (NCNM) has a give-away economy with no energy overhead and with the same income for everyone whether they work or not.  It is most like the natural economy advocated in On the Preservation of Species, “Energy in a Natural Economy”, “On the Conservation-within-Capitalism Scenario”, and “The Demise of Business as Usual” all of which are hyperlinked to http://dematerialism.net/.

The two levels of conservation are as follows with the second level split:

1.         Conservation Level 1 is no conservation at all or rather only such conservation measures as have been implemented in the US American economy at the present time.

2.         Conservation Level 2 is any conservation factor, ψ, less than 1.0 in the linear relations that adjust the levels of consumption of the four commodities between their values in the Base Case, which represents the US economy at the present time, and a fraction φ_i of the present value where φ_A = 0.2, φ_R = 0.3, φ_M = 0.1, and φ_T = 0.1; and that adjusts the energy overhead for each of the four sectors C, A, M, and, T between their values for the Base Case and a fraction ξ_i of the present value where ξ_C = 0.5, ξ_A = 0.1, ξ_M = 0.5, ξ_T = 0.1.

2a.       With an EROI^o = 21 for a fossil-fuel economy, the conservation factor ψ is reduced until the energy budget in the Base Case has been reduced to Pimentel’s value for Maximum Renewables. 

2b.       With an EROI^o = 3 for a renewable-energy economy, the conservation factor has been reduced until the energy budget for the No-Commerce-No-Managers (NCNM) Case corresponding to the Natural Economy has been reduced to Maximum Renewables.

The final EROI calculations are done on Sheet 4 in the block spanned by DN48 and DR66.  The principal computations are in Columns GM through IV, the end of the spreadsheet.

The Effect of Lowering EROI toward 1.0.

This computation was performed and tabulated for the Base Case on Sheet 4 in the block spanned by DN100 and DS118.  Here is the block from Sheet 4 of Mark-II-Economy-CSP.xls:

 

 

The results in DT100::DU118 are plotted in Chart 2 on its own sheet; however, the chart on the cover page comes from an earlier calculation and has been displayed there for aesthetic reasons.  (Also, it includes the behavior of the GDP when EROI is lowered.)  Here is Chart 2 from Mark-II-Economy-CSP.xls:

 

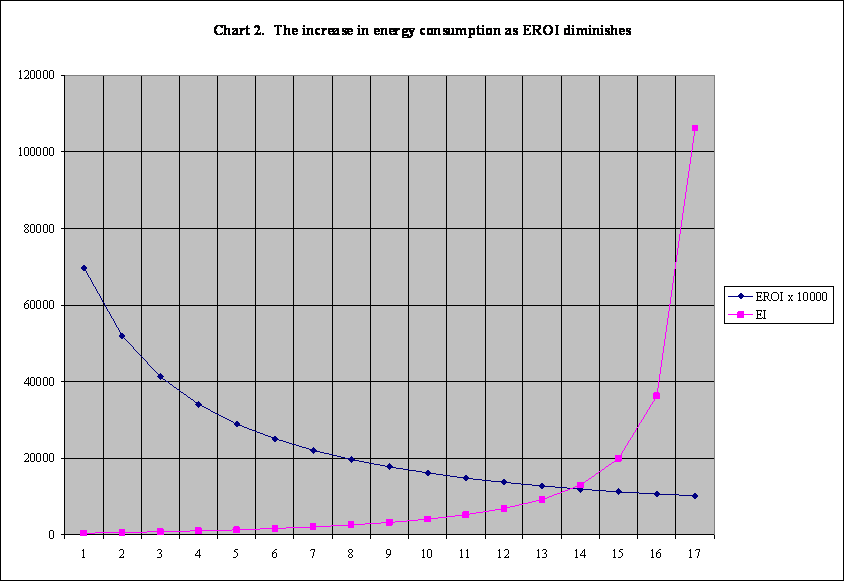
The spreadsheets have only 711 rows; therefore, they are not truly converged for the lowest EROIs.  Even Cell 710 reports a substantial contribution to EI.  When I tried to converge the spreadsheet for eighteen times the direct energy overhead for the delivery of one energy unit, Newton’s method diverged and extremely large numbers were encountered immediately.  The sequence of contributions to EI increased from Cell FJ706 to Cell FJ710.  Well before this happened, though, the production required of the various sectors of the economy became infeasible.

The numbers in Column DS above are for Payback Time (PT) Factor.  This is the ratio of the actual payback time computed from EROI⁴ to the payback time that would have been calculated if the purveyor of the energy technology in question reported EROI^o instead, which, if it were ever done, and I am not claiming that it has ever been done, would have constituted a false claim.

Thomas L Wayburn

Houston, Texas

October 16, 2006